Want more ideas and freebies?
Get my free resource library with digital & print activities—plus tips over email.
Join for free resources →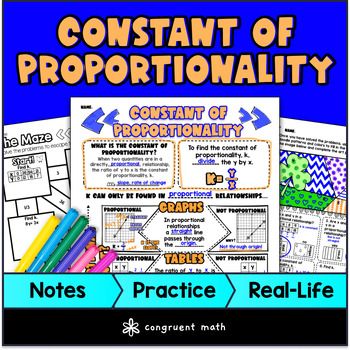Get the Lesson Materials
Constant of Proportionality Guided Notes & Doodles | Proportional Relationships
$4.25
Overview
Ever wonder how to teach constant of proportionality to your students? Looking for ideas to make it relevant to your students' life?
Use this artistic, real-life lesson plan to teach your students about the constant of proportionality and how to find k from graphs, equations, and tables.
Students will learn how constant of proportionality explains why their cell phone charger gets so hot, and why different devices take different amounts of time to charge. This lesson plan introduces the topic with guided notes (like sketch notes), practices the topic with a doodle & color by number worksheet and a maze activity, and wraps up with the real life application of constant of proportionality for cell phone chargers.
- Standards: CCSS 7.RP.A.2.a, CCSS 7.RP.A.2.b
- Unit: 7th Grade → Unit 4: Ratios, Rates & Proportions
- Grade: 7th Grade
Get the Lesson Materials
Constant of Proportionality Guided Notes & Doodles | Proportional Relationships
$4.25
Learning Objectives
After this lesson, students will be able to:
Identify proportional relationships in tables, graphs, and equations.
Determine the constant of proportionality (k) for a given proportional relationship.
Apply the concept of constant of proportionality to real-life scenarios, such as cell phone chargers and other electronic devices that require charging.
Prerequisites
Before this lesson, students should be familiar with:
Ratios and proportions
Graphing on a coordinate plane
Equivalent ratios and their relationship to proportional relationships
Materials
Pencils
Colored pencils or markers
Constant of Proportionality Guided Notes
Key Vocabulary
Proportional relationship
Constant of proportionality
Unit rate
Equivalent ratios
Coordinate plane
Graph
Procedure
Introduction
As a hook, ask students why your cell phone charger gets so hot, or how different devices plugged into the same charger take different amounts of time to charge. Refer to the last page of the guided notes as well as the FAQs below for ideas.
Use the guided notes to introduce the concept of proportional relationships and the constant of proportionality. Refer to the FAQ below for a walk through on this, as well as ideas on how to respond to common student questions.
Walk through how to determine whether something is a proportional relationship and what the constant of proportionality is in tables, graphs, and equations.
Check for Understanding
Have students work through the problems on the check for understanding page, either collaboratively or independently.
Walk around and spot-check student answers on the check for understanding activity.
Based on student responses, reteach concepts that students need extra help with. If your class has a wide range of proficiency levels, you can pull out students for reteaching, and have more advanced students begin work on the practice exercises.
Practice
Have students practice finding the constant of proportionality using the Doodle Math activity. Walk around to answer student questions.
Fast finishers can dive into the maze activity for extra practice. You can assign it as homework for the remainder of the class.
Real-Life Application
Bring the class back together, and introduce the concept of how the constant of proportionality impacts how hot their cell phone charger gets and how fast it charges. Refer to the FAQ for more ideas on how to teach it, including a video!
Extensions
Additional self-checking digital practice
If you’re looking for digital practice for constant of proportionality, try my Pixel Art activities in Google Sheets. Every answer is automatically checked, and correct answers unlock parts of a mystery picture. It’s incredibly fun, and a powerful tool for differentiation. There’s fall, winter, and spring versions perfect for additional practice.
Additional print practice
A fun, no-prep way to practice constant of proportionality is Doodle Math — it's a fresh take on color by number or color by code. It includes 3 levels of practice, and there’s fall and winter themed versions, perfect for a review day or sub plan around the holidays.
FAQs
A proportional relationship is a relationship that exists between two variables where one variable is a constant multiple of the other. This means that as one variable changes, the other variable changes in a predictable way.
On a graph, a proportional relationship will be represented by a straight line that passes through the origin.
On a table, a proportional relationship will have a constant ratio between the two variables after you simplify.
In an equation, a proportional relationship will have a constant of proportionality (k) that relates the two variables through the equation y = kx.
Understanding proportional relationships is important in many areas of math and science, as well as in real life situations where one variable depends on another in a predictable way.
The constant of proportionality is the ratio that exists between two related variables in proportional relationships and can be found in graphs, tables, and equations.
On a graph, we can determine the constant of proportionality by studying the slope.
On a table, we can determine the constant by looking at the ratio of the two variables after you simplify.
And from an equation, we can find the constant, k, by rearranging the equation into the format k = y/x.
It is important to practice all three methods to help students understand the connections between them.
Yes, the constant of proportionality is the same as slope when there is a proportional relationship (i.e. the straight line goes through the origin).
Yes, the constant of proportionality is the unit rate when one of the quantities is measured in "1" units. For example, 63 meters/second has k = 63.
Yes, the constant of proportionality is the same as the scale factor because they both represent the ratio between two related variables, and can be used interchangeably.
Yes, the constant of proportionality can have units. For example, if a proportional relationship is between distance and time, the constant of proportionality might have units of meters per second.
Yes, the constant of proportionality can be negative, when the quantities in the relationship are inversely proportional, meaning that as one quantity increases, the other decreases. In this case, the constant of proportionality would be a negative number.
Yes, the constant of proportionality can be 0. This would occur when the two quantities in the proportional relationship are not actually proportional. In this case, there would be no constant factor relating the two quantities, so the constant of proportionality would be 0.
On a graph, we can determine the constant of proportionality by studying the slope. For students that need a visual explanation, I find this Khan Academy video helpful:
On a graph, we can determine the constant of proportionality by studying the slope. For students that need a visual explanation, I find this Khan Academy video helpful:
In an equation, we can find the constant, k, by rearranging the equation into the format k = y/x. For students that need a visual explanation, I find this Khan Academy video helpful:
The constant of proportionality is used everywhere in everyday life, including when you charge your phone. The YouTube personality MKBHD made this video about the science of cellphone charging, and the first 5 minutes highlights multiple constant of proportionality problems:
(As always, make sure to watch the video in advance to ensure it's grade-appropriate.)
Here's the places I saw constant of proportionality in the video:
How fast will my cell phone charge? When a cellphone is connected to a charger, there is a direct relationship between the amount of energy that is drawn from the charger and the rate at which the device will be charged. In other words, if the device is drawing more watts of electricity, the device will charge faster. This relationship is the constant of proportionality
How hot will the charger will get? MKBHD mentioned that there's inefficiency in chargers at certain points in the charging cycle. If the device isn't using all the energy that is being provided by the charger, the excess energy will convert to heat and make the charger hot. This heat is a byproduct of the constant of proportionality which explains why your phone charger gets so hot.
How long will my battery last? And as your phone gets older, as MKBHD discussed, your battery degrades. There's a relationship between the cellphone degradation number in you phone setting, and how many hours your phone will last for between charges.
So next time you're looking at your phone charger, remember that the constant of proportionality is at work—keeping your device charged and the charger cool.