Want more ideas and freebies?
Get my free resource library with digital & print activities—plus tips over email.
Join for free resources →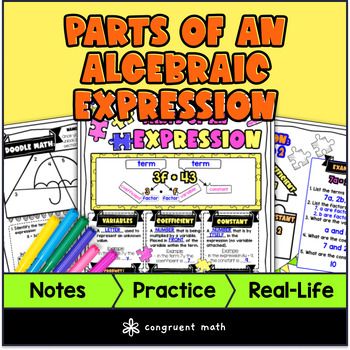Get the Lesson Materials
Identifying Parts of an Expression Guided Notes with Doodles | Sketch Notes
$4.25
Overview
Looking for way to teach identifying the parts of an expression with 6th or 7th grade students?
Use this artistic, real-life lesson plan to teach your students about identifying parts of an algebraic and numerical expression. It covers coefficients, variables, constants, terms and operations. Students will learn material with artistic guided notes (interactive sketch notes), check for understanding, and practice with a doodle and color by number activity, and a maze activity.
It concludes with the real-life application of shopping cart subtotals, covering how expressions are used to total up the contents of the cart, calculate shipping, and more.
- Standard: CCSS 6.EE.A.2.b
- Unit: 6th Grade → Unit 5: Algebraic Expressions
- Grade: 6th Grade
Get the Lesson Materials
Identifying Parts of an Expression Guided Notes with Doodles | Sketch Notes
$4.25
Learning Objectives
After this lesson, students will be able to:
Identify the parts of an expression (terms, coefficients, variables, and constants)
Understand how parts of an expression relate to real-life situations, such as online shopping carts
Note: This will cover breaking down an expression into parts, not writing or evaluating an expression. Check out my lesson plans for those!
Prerequisites
Before this lesson, students should be familiar with:
Basic arithmetic operations such as addition, subtraction, multiplication, and division
Order of operations
Materials
Pencils
Colored pencils or markers
Identifying Parts of Expressions Guided Notes
Key Vocabulary
Expression
Term
Coefficient
Variable
Constant
Procedure
Introduction
As a hook, ask students how a shopping cart decides how much an order costs. Explain that parts of expressions are critical for powering this. Refer to the FAQs for ideas.
Use the guided notes to introduce the different parts of an expression, such as terms, coefficients, variables, and constants. Walk through the example expression on the second guided notes page together. Refer to the FAQs below for ideas on how to respond to common student questions.
Check for Understanding
Have students work through the problems on the “You Try!” check for understanding page, either collaboratively or independently.
Have students color the responses in the check for understanding section of the second page. Call on students to talk through their answers, potentially on the whiteboard or projector. Based on student responses, reteach concepts that students need extra help with.
Walk around and spot-check student answers on the check for understanding activity.
Based on student responses, reteach concepts that students need extra help with. If your class has a wide range of proficiency levels, you can pull out students for reteaching, and have more advanced students begin work on the practice exercises.
Practice
Have students work through the maze activity to identify the parts of different expressions. Walk around to answer student questions and offer guidance.
For fast finishers, have them work on the Doodle Math activity to reinforce their understanding of the parts of an expression.
Real-Life Application
Bring the class back together, and introduce the concept of calculating subtotals in a shopping cart. Explain how understanding the parts of an expression can help us understand how shopping carts work, and how expressions are used to calculate the total cost of items in a cart. Refer to the FAQs for more ideas on how to teach it!
Extensions
Real-Life Application Project
Have students research and calculate the cost of an online shopping cart. They should select items from a real online store and add them to their cart. Once the items are added, students should calculate the cost of the items, any applicable taxes, and shipping fees. They should also identify the different parts of the expression used to calculate the total cost of the order.
As an alternative, have students create their own online store and determine the cost of items in their own shopping cart. They should create a list of items with prices and assign values to variables such as tax rates and shipping fees. Once they have created their own store and items, they should calculate the total cost of an order using the expression they have created.
FAQs
Algebraic expressions are mathematical statements that involve variables, constants, and arithmetic operations such as addition, subtraction, multiplication, and division. They are used to represent real-world situations and can be manipulated and simplified using algebraic rules and properties.
The parts of an expression include terms, coefficients, variables, and constants.
A term is a single numerical or algebraic expression separated by addition or subtraction operators within an overall expression. For example, in the expression 3x + 2y - 5, 3x, 2y, and -5 are all individual terms.
A coefficient is the numerical factor that is multiplied by a variable in an algebraic expression. For example, in the expression 3x + 2y - 5, the coefficient of x is 3, and the coefficient of y is 2.
A variable is a symbol that represents a quantity that can change or vary. For example, in the expression 3x + 2y - 5, x and y are variables that can be replaced with specific numbers to produce a value for the expression.
A constant in an expression is a fixed numerical value that does not change. It is not multiplied by a variable, and it does not have any variables added or subtracted from it. For example, in the expression 3x + 2y - 5, -5 is a constant.
To identify the parts of an expression, follow these steps:
Look for terms: A term is a numerical or algebraic expression separated by addition or subtraction operators within an overall expression.
Identify coefficients: The coefficient is the numerical factor that is multiplied by a variable in an algebraic expression.
Identify variables: A variable is a symbol that represents a quantity that can change or vary in an expression.
Identify constants: A constant is a fixed numerical value that does not change and is not multiplied by a variable.
If your students need a more visual example, here’s a pair of helpful Khan Academy videos:
Algebraic expressions are highly applicable in various fields, here are some specific examples:
Finance: Algebraic expressions can be used to calculate costs, revenue, and profit in a business. For example, a business owner can use algebraic expressions to determine the cost of producing goods or services, the revenue from sales, and the profit margin.
Engineering: Engineers use algebraic expressions to design and analyze structures such as bridges and skyscrapers. For instance, they can use algebraic expressions to calculate the load capacity of a bridge and ensure its stability.
Physics: Algebraic expressions are commonly used in physics to model and solve problems related to motion, energy, and forces. For example, algebraic expressions can be used to calculate the velocity of an object, the amount of work done on an object, and the force exerted on an object.
Computer Science: Computer scientists use algebraic expressions to write algorithms and perform various computations. For instance, they can use algebraic expressions to calculate the running time of an algorithm or to represent data structures such as arrays and matrices.
Statistics: Algebraic expressions are used in statistics to analyze data and make predictions. For example, algebraic expressions can be used to calculate the mean, median, and mode of a set of data, as well as to calculate probabilities and make predictions based on statistical models.
Expressions are used to calculate the subtotals for online shopping carts.
For example, consider a shopping cart with two board games, one priced at $25 and the other priced at $30.
The subtotal expression for this cart would be
25 + 30.In this expression,
25and30are the terms, and+is the operation that combines the terms.
By identifying the parts of this expression, such as the terms and operation, we can better understand how the expression works and how changes to the cart affect the subtotal.