Want more ideas and freebies?
Get my free resource library with digital & print activities—plus tips over email.
Join for free resources →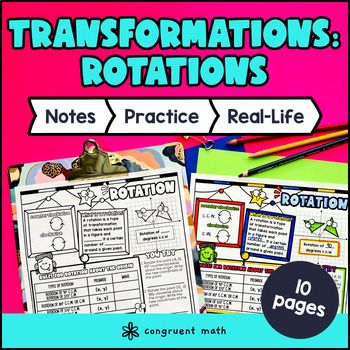Get the Lesson Materials
Rigid Transformations Rotations Guided Notes & Doodles | 8th Grade Geometry
$4.25
Overview
Ever wondered how to teach rotations in an engaging way to your 8th grade geometry students?
In this lesson plan, students will learn about rotations and their real-life applications. Through artistic and interactive guided notes, check for understanding questions, a doodle & color by number activity, and a maze worksheet, students will gain a comprehensive understanding of rotations.
The guided notes provide structured information on the rules for rotations about the origin for 90, 180, and 270 degrees, as well as graphing rotations of figures. These notes integrate checks for understanding to ensure students are on the right track.
After reviewing the guided notes, students will apply their understanding through a practice worksheet that includes a color by code activity, a maze, and problem sets. This hands-on activity will engage students and help them solidify their knowledge of rotations.
To further connect rotations to real-life situations, students will read and write about the real-life uses of rotations. This application activity will help students see the relevance and practicality of the topic.
By the end of this lesson, students will have a solid understanding of rotations and how they can be applied in real-life situations. They will also have developed their skills in graphing figures on coordinate planes after rotations about the origin, understanding counterclockwise and clockwise rotations, writing rules for transformations when given graphed figures, and writing coordinate points for preimages and images of figures undergoing rotations.
- Standard: CCSS 8.G.A.3
- Unit: 8th Grade → Unit 5: Transformations
- Grade: 8th Grade
Get the Lesson Materials
Rigid Transformations Rotations Guided Notes & Doodles | 8th Grade Geometry
$4.25
Learning Objectives
After this lesson, students will be able to:
Identify and describe rigid transformations, specifically rotations, including rotations of 90, 180, and 270 degrees about the origin
Graph figures on coordinate planes after rotations about the origin
Determine the rules for transformations when given graphed figures undergoing rotations
Write coordinate points for preimages and images for figures undergoing rotations
Prerequisites
Before this lesson, students should be familiar with:
The coordinate plane and plotting points on it
Materials
Pencils
Colored pencils or markers
Key Vocabulary
Rigid transformations
Rotations
Origin
Counterclockwise
Clockwise
Preimage
Image
Procedure
Introduction
As a hook, ask students why rotations are important in real life applications. For example, ask them how rotations are used in video games to move characters or objects. Refer to the last page of the guided notes for a more detailed example of how rotations are used in jet engines.
Use the first page of the guided notes to introduce rotations about the origin for 90, 180, and 270 degrees. Walk through the rules for each rotation and discuss the effects of rotating figures. Emphasize the concept of counterclockwise and clockwise rotations. Use the practice problems of the guided notes to introduce graphing figures after rotations about the origin. Explain how the coordinates of each point change after the rotation and give examples using different figures.
Based on student responses, reteach concepts that students need extra help with. If your class has a wide range of proficiency levels, you can pull out students for reteaching, and have more advanced students begin work on the practice exercises.
Practice
Have students practice rotations using the practice worksheet provided in the resource on page 2. This includes graphing figures that undergo rotation about the origin, and writing coordinate points of preimage and image.
Then, they can complete the color by code, maze, and problem sets to reinforce their understanding of rotations (pg. 3 and 4 of the guided notes).
Walk around the classroom to answer any student questions and provide assistance as needed.
Real-Life Application
Bring the class back together, and introduce the concept of real-life applications of rotations. Rotations are used in various fields such as architecture, engineering, and computer graphics. In computer graphics, rotations are used to create animations and special effects in movies and video games. Have students read the last page of the guided notes and reflect on real life uses of rotations.
Refer to the FAQ for more ideas on how to teach the real-life applications of rotations and engage students in relating this mathematical concept to the world around them.
Extensions
Additional Self-Checking Digital Practice
If you’re looking for digital practice for reflections, try my Pixel Art activities in Google Sheets. Every answer is automatically checked, and correct answers unlock parts of a mystery picture. It’s incredibly fun, and a powerful tool for differentiation.
Here’s a reflection activity to explore:
Additional Print Practice
A fun, no-prep way to practice rotations is with the Doodle Math activities. They provide a fresh take on color by number or color by code. It includes multiple levels of practice, perfect for a review day or sub plan.
Here are some activities to try:
Transformations Reflections, Rotations, and Translations Doodle Math
FAQs
Rigid transformations are transformations that preserve the size, shape, and orientation of a figure. They include translations, reflections, and rotations.
A rotation is a transformation that turns a figure around a fixed point called the center of rotation. The figure is rotated a specific number of degrees either clockwise or counterclockwise.
To perform a rotation of 90 degrees counterclockwise about the origin, switch the x and y coordinates of each point and then negate the new x coordinates.
To perform a rotation of 180 degrees about the origin, simply negate both the x and y coordinates of each point.
To perform a rotation of 270 degrees clockwise about the origin, switch the x and y coordinates of each point and then negate the new y coordinates.
To graph a figure after a rotation about the origin, apply the rotation rule to each point of the figure. Visualize the rotations and plot the new points on the coordinate plane.
To write the rule for a rotation about the origin, simply determine the new coordinates of each point after the rotation. The rule will specify how the x and y coordinates are transformed.
Rotations have various real-life applications, such as:
In computer graphics, rotations are used to create animations and special effects in movies and video games.
Designing symmetrical patterns in art and architecture