Want more ideas and freebies?
Get my free resource library with digital & print activities—plus tips over email.
Join for free resources →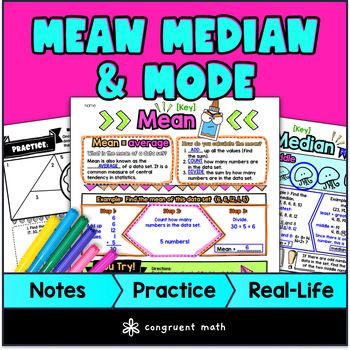Get the Lesson Materials
Mean Median Mode Guided Notes & Doodles | Measures of Central Tendency
$4.25
Overview
Have you ever wondered how to teach mean median and mode in a fun way to your 5th and 6th grade students?
In this lesson plan, students will learn about measures of central tendency and their real-life applications. Through artistic, interactive, guided notes, a doodle & color by number worksheet, and a maze activity, students will gain a comprehensive understanding of mean, median, and mode.
The lesson culminates in a real life example that explores how sports analysts decide which players are performing the best, and which should be replaced based on calculations.
- Type: Lesson Plans
- Grade: 6th Grade
- Standards: CCSS 6.SP.B.5.c, CCSS 6.SP.A.3
- Duration: 2 Hours
- Topic: Statistics & Probability
Get the Lesson Materials
Mean Median Mode Guided Notes & Doodles | Measures of Central Tendency
$4.25
Learning Objectives
After this lesson, students will be able to:
Define mean, median, and mode
Calculate mean, median, and mode from a given set of data
Understand the application of mean, median, and mode in a real-life application, specifically sports analytics in the context of baseball
Note: This lesson plan doesn't cover range. See "extensions" for ideas!
Prerequisites
Before this lesson, students should be familiar with:
Basic math operations (addition, subtraction, multiplication, and division)
Understand how to arrange numbers in numerical order
Comparing numbers
Materials
Pencils
Colored pencils or markers
Mean, Median, Mode Guided Notes
Key Vocabulary
Mean
Median
Mode
Measures of central tendency
Outliers
Data set
Procedure
Introduction
As a hook, ask students if they have ever wondered how sports analysts calculate which players are performing the best and which should be replaced. Explain that measures of central tendency, specifically mean, median, and mode, are used to make these decisions.
Introduce the learning objectives for the lesson plan.
Use the guided notes to introduce mean and how to find it in a data set. Refer to the FAQ below for a walk through on this, as well as ideas on how to respond to common student questions.
Check for Understanding. Have students walk through the “You Try!” section. Call on students to talk through their answers, potentially on the whiteboard or projector. Based on student responses, reteach concepts that students need extra help with.
Use the guided notes to introduce median and how to find it in a data set. Refer to the FAQ below for a walk through on this, as well as ideas on how to respond to common student questions.
Check for Understanding. Have students walk through the “You Try!” section. Call on students to talk through their answers, potentially on the whiteboard or projector. Based on student responses, reteach concepts that students need extra help with.
Use the guided notes to introduce mode and how to find it in a data set. Refer to the FAQ below for a walk through on this, as well as ideas on how to respond to common student questions.
Check for Understanding. Have students walk through the “You Try!” section. Call on students to talk through their answers, potentially on the whiteboard or projector. Based on student responses, reteach concepts that students need extra help with.
Practice
Have students practice finding mean, median, and mode using the maze activity. Walk around to answer student questions.
Fast finishers can dive into Doodle Math maze activity for extra practice. You can assign it as homework for the remainder of the class.
Real-Life Application
Bring the class back together, and introduce the concept of how mean, median, and mode are used in sports analytics, with different sports statistics the correspond to different statistics. Refer to the FAQ for more ideas on how to teach it!
Extensions
Additional Self-Checking Digital Practice (Pixel Art Google Sheets)
If you’re looking for digital practice for mean, median, and mode, try my Pixel Art activities in Google Sheets. Every answer is automatically checked, and correct answers unlock parts of a mystery picture. It’s incredibly fun, and a powerful tool for differentiation. There’s Easter, Valentine’s Day, and year-round Minecraft-themed versions.
Challenge with Range
If you’re looking for practice problems for range, in addition to mean, median, and mode, try my Doodle Math print activity, and Domino Effect collaborative math game.
FAQs
A data set is a collection of numerical values or other data points.
Outliers are data points that are significantly different from the rest of the data.
Measures of central tendency are statistical values that represent the center or middle of a data set. Mean, median, and mode are examples of measures of central tendency.
Mean, median, and mode are all measures of central tendency used in statistics.
Mean is the average of the data set, calculated by adding up all the values in a data set and dividing by the number of values.
Median is the middle value in a data set when the values are arranged in order.
Mode is the value that occurs most frequently in a data set.
Mean is the average, median is the middle, and mode is the most frequent.
Mean, median, and mode are calculated in slightly different ways:
To calculate the mean, add up all the values in the data set and divide by the total number of values.
To calculate the median, arrange the values in order and find the middle value. For an even number of values, take the average of the two middle values.
To find the mode, determine which value occurs most frequently in the data set.
If you have a student that needs a video explainer, here’s a helpful Khan Academy video:
In the context of sports analytics, mean, median, and mode can be used to evaluate player performance based on statistics like on base percentage, batting average, and wins above replacement.
Batting average is a statistic used in baseball to measure a player's performance at the plate. It is calculated by dividing the number of hits a player has by the number of times they have at-bats. In statistics, batting average is an example of mean, which is a measure of central tendency used to represent the center or middle of a data set.
On base percentage (OBP) is a baseball statistic that measures how often a player reaches base. It is calculated by dividing the total number of times the player has reached base (hits, walks, hit by pitch) by the total number of plate appearances (at-bats, walks, hit by pitch, sacrifice fly). While OBP is technically an example of mean in statistics, as it represents the average rate at which a player reaches base. However, like median, it is less affected by outliers than mean. For example, if a player has an OBP of .500 but only gets on base in one of their ten plate appearances, their median would be .100, whereas their mean would be .500.
Wins above replacement (WAR) is a baseball statistic that measures a player's value in comparison to a "replacement level" player. A replacement level player is defined as a player who is readily available to be signed from the minor leagues or free agency. WAR is calculated by comparing a player's performance to that of a replacement level player, and then adjusting for the player's position and the park in which they play.
In statistics, WAR is an example of a measure of central tendency called mode, which represents the most common or frequent value in a data set. In the case of baseball, a player's WAR is often used to determine their overall value and contribution to their team.